1 − 2 + 3 − 4 + ⋯

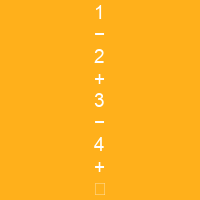
In mathematics, 1 − 2 + 3 − 4 + ··· is an infinite series whose terms are the successive positive integers, given alternating signs. In 1891, Ernesto Cesàro expressed hope that divergent series would be rigorously brought into calculus, pointing out that both the sides are equal to ​1⁄4.

About 1 − 2 + 3 − 4 + ⋯ in brief

In mathematics, 1 − 2 + 3 − 4 + ··· is an infinite series whose terms are the successive positive integers, given alternating signs. Using sigma summation notation the sum of the first m terms of the series can be expressed asThe infinite series diverges, meaning that its sequence of partial sums,, does not tend towards any finite limit. In 1891, Ernesto Cesàro expressed hope that divergent series would be rigorously brought into calculus, pointing out that both the sides are equal to ​1⁄4. The Cauchy theorem is the first application of this theorem in which the product of two infinite series is defined when both of them are divergent. In the case where an n = n, the central idea is that 1 + 3 + 4 +… is the Product of 1 − 1 + 1. + 1.. with the terms of 1. −2, 3, −4, 5, −6, follow a simple pattern. The series’ terms do not approach 0; therefore 1-2 + 3-4-4 diverges by the term test. Although the series does not have a sum in the usual sense, it can be manipulated by shifting and term-by-term addition to yield a numerical value. So s=14, which is the most natural answer if such a sum is to be defined. For later reference, it will also be useful to see the divergence on a fundamental level.

By definition, the convergence or divergence of an finite series is determined by the convergence of its partial sums. This sequence is notable for including every integer exactly once, and thereby establishing the countability of the set Z{displaystyle mathbb {Z} } of integers. It is also notable that every integer in the sequence is counted even if it is empty, such as 0 if one counts the empty partial sum. The details on his summation method are below; the main idea is to show that the terms are even when an n is an n, with the product of n + b = 1 + b + 1 + 2 + 1 with the term b = b + b. The summability method is a linear and stable way to assign generalized sums to divergentseries, and it is desirable that they share certain properties with ordinary summation. The method is called a summation methods or summability methods. In 1890, Cesào expressed his hope that Divergent Series would be brought in to calculus. He already writes 2 + 2. + 3. + 4n + n + n = 1- 2n + 3n − 4n. + n for arbitrary n, a line of research leading towards the functional equations of what are now known as the Dirichlet eta function and the Riemann zeta function.

Related posts:

Main sequence Scientific method Euclidean algorithm Infinite Jest